Which is better: many short exposures or fewer long exposures? 120 x 1 minute exposures or 10 x 12 minute exposures? Questions similar to this one get asked really often and they probably receive all kinds of different answers. People talk about the camera, faintness of the signal, dithering, stacking, light pollution etc. etc. So many factors creep into the discussion that sooner or later it will seem like a personal choice, almost like a matter of opinion. Is this really a matter of opinion and/or complicated? Or can we find some definitive answer to the question if more shorter exposures are better than fewer longer exposures?
Actually, it turns out that although there are formulas for ideal (theoretical!) exposure times, personal preferences and opinion and practicality will come into play in reality when determining the exposure you want to be using.
Knowing how the subexposure time will impact the SNR of your stacked image will help you to determine the exposure time you choose to use. Please bear with me while we explore the way to determine this optimum exposure time, which will include quite a bit of math. I find that going over the math and actually calculate the SNR for a few different scenarios helps greatly in my understanding of this matter. So I encourage you to follow along with the math. For those who are mostly interested in the key take aways I’ll start with those in a short summary, before diving into details and the math further on.
Key take aways
When you are imaging under light polluted skies, you should not worry about your exposure times. It will make very little to no difference if you are using subexposures of 30sec. or 3minutes. It’s the total integration time that matters.
If you are imaging under dark skies, you will benefit from longer exposures in terms of SNR. However, it’s not worth it to go to extremes and keep in mind the cost of throwing out a subexposure due to poor tracking for instance. Only increase exposure time if you can reliable track accurately for that long.
If you don’t use darks and/or want to use dithered (bayer) drizzel integration, make sure you get at least 10 to 15 subexposures.
Now that we’ve learned not to worry about subexposure time under light polluted skies and give subexposure time priority under dark skies, let’s see why this is the case and how we arrive at these conclusions.
Read noise and background sky flux
To determine the optimum exposure it basically comes down to these two factors; read noise and the background sky flux.
The read noise is the noise that occurs within the electronics of the camera when we convert the electrons coming from the sensor into digital units (ADU) and store them. The background sky flux is the signal coming from the light pollution, moon light and/or air glow. The background is never completely black and the value of the background signal is the background sky flux.
If the read noise is swamped by sky background noise it becomes irrelevant and it doesn’t matter how long our subexposures are
If we wouldn’t have read noise, it wouldn’t matter if we take one very long exposure or many shorter exposures with the same total integration time. If we wouldn’t have background sky noise but do have read noise, the longer exposure would always have better SNR than many shorter exposures.
In reality we always have to deal with some sort of mixture of these two scenarios. There will always be some background sky noise and read noise is always a factor to take into account. Especially for us DLSR users. So how should we take those two factors into account? Well, it comes down to determining the point where the sky background noise will make the read noise irrelevant. Whenever this is the case, it doesn’t matter any more if we take many shorter or fewer longer exposures.
So if you are imaging under strong light pollution this probably applies to you. Alternatively, if you are using narrowband imaging and/or if you are under truly dark skies, it will be practically impossible to reach this point and so the longer exposures will always be better.
Now let’s research why this is the case and look at some test results;
Adding multiple exposures and SNR
Let’s look at what exactly happens to the SNR when we add multiple exposures and when we expose longer. We will dive into some math here and I will try to do this step by step and as clear as possible. I know lot’s of articles will skip steps and rewrite formulas without explaining how or why which I’ll try to avoid.
The SNR is simply the Signal divided by the noise. Sounds simple, but with different noise sources we need to dive in a bit deeper and see how we add noise. But first let’s define the term Signal exactly: S = signal per second (s) * time of the exposure (t) * number of exposures (N). So
To be clear; this is the total amount of signal recorded over multiple exposures.
Next let’s consider the noise sources we are dealing with here: Object shot noise, sky (shot) noise, dark current noise and read noise. Whenever we are dealing with detecting photons we deal with shot noise. This shot noise is the square root of the number of photons. This noise is also building up over time just like the signal. So in terms of the object shot noise, it is the noise associated with the signal of the object(s) we are imaging and is the square root of the signal.
For sky shot noise it is the square root of the background signal coming from the sky over time.
For the dark current noise it is the square root of the thermal signal (dark current) build up.
The read noise is different in the sense that this doesn’t build up over time but it does occur once for every exposure. This is important to realise. The total read out noise (RonTot) is:
We can sum random, uncorrelated noise by adding them quadratically so we get the following formula for the total amount of noise:
Remember the fact that the shot noise is the square root of the Signal source. So if we add this quadratically we can rewrite the Noise in terms of the signal like this:
Since the number of exposures (N) is present in each term we can rewrite this as:
or
So the formula for the SNR is:
Notice we have N as a term present in both parts of the division. Since we can rewrite the above formula into:
Now we have written the formula down for SNR in this useful format, we can explore what the impact of the background sky flux and the read noise is on the SNR.
Scenario with no read noise
Maybe you start to see now what we said earlier; if we wouldn’t have read noise it wouldn’t matter how long we expose as long as the total exposure time is the same. Let’s just take out the read noise (RN) out of the above equation. Without read noise the SNR will be:
Since we have t present now in every term we can group it:
which is the same as
and as we’ve seen before we can write this down as
Now we can see it doesn’t matter for the SNR how we fill in values for N and t as long as N*t = the same value. So only total exposure time matters (N*t) and not how we divide it in subexposures.
But wait, what about really faint signal? Don’t you need very long exposures for that? Well, not in this case where we don’t have read noise. You just need (very) long total exposure time. If we look at the above formula this is clear, but if we think of stacking and consider that we are averaging there this might seem less intuitive all of a sudden. Because, the average of a few electrons collected by many frames is smaller than the average of a few electrons in one frame right? Well, sure that is correct of course. However, this is only considering signal. And it is not useful to talk about signal alone, we always need to talk about SNR. That alone determines if you will have detected signal that will stand out in an image or not.
And if we consider the fact that SNR = simply signal / noise you’ll realise it doesn’t matter in how many frames we detected the signal, since we’ll only end up dividing both terms of the division which doesn’t change anything ((a*b)/(a*c) = (a/a) * (b/c) = 1*(b/c) = (b/c))
So yes, it’s true that if read noise wouldn’t exist it doesn’t matter what exposure time you use and how many exposures you take, all that matters is the total integration time. And even with read noise included in the formula, you can see that once the other values are much much bigger than the read noise, the same will apply; the read noise becomes (almost) irrelevant and we are left in the situation where it doesn’t matter what exposure time you use.
once the other noise values are much much bigger than the read noise, the read noise becomes (almost) irrelevant and we are left in the situation where it doesn’t matter what exposure time you use.
Scenario with little to no sky noise
Alternatively, consider the scenario where we are under a truly dark sky with no light pollution, no moon light and only a little sky glow; we have very little background sky signal (Sky_s). Let’s consider the SNR formula again:
Now let’s say the DarkCurrent_s = 0.15e-/sec (which I found reported for the Nikon D7000), Sky_s = 1e- / sec and read noise = 3e- (Nikon D7000 @ ISO200). If we take a very faint signal that’s similar to the sky flux we will see the following SNR values for different number of exposure times but same total exposure time:
Let’s compare scenarios with a total integration time of 120 minutes and compare 120x1min and 12x10min.
120x1min:
12x10min:
Ok, so SNR is higher indeed as expected. What if we take this to the extreme and just take 1 image of 120min?
1x120min:
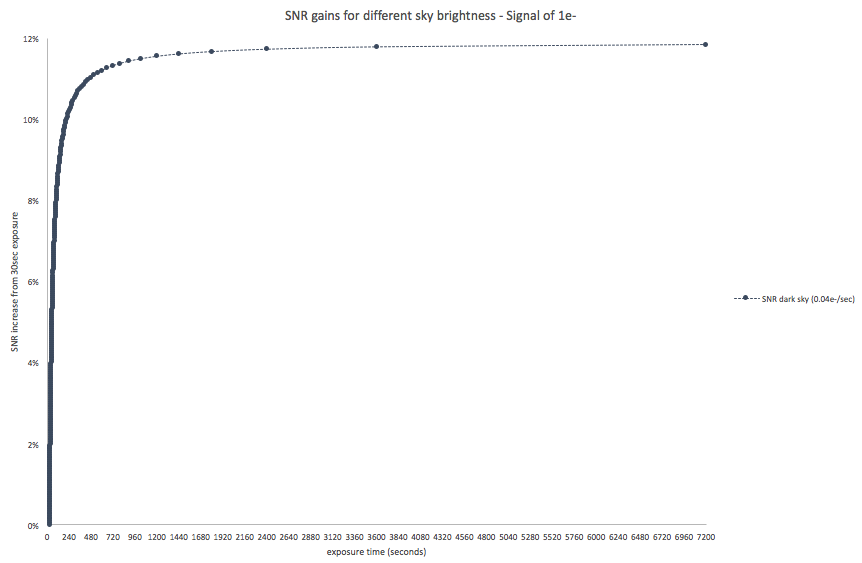
Hmmm, that’s a really small improvement over the 12x10min exposure. Clearly this is a case of (quickly) diminishing returns. I made a graph showing the SNR gains compared to a 30sec exposure SNR for exposures between 30sec and 120min to show the benefit of exposing longer in this scenario:
This graph paints quite a clear picture I’d say. In case of a dark sky, the gains in SNR while exposing longer is quite big in the beginning and reaches a 10% improvement already at 3,5minutes exposures compared to 30sec exposures. The improvements tail off quickly as well though, reaching only a further 1% improvement at 8 minutes compared to the 30sec exposures. To be clear; in this scenario, the difference between exposing 3.5minutes and 8 minutes (with the same total integration time) is even slightly less than 1% improvement.
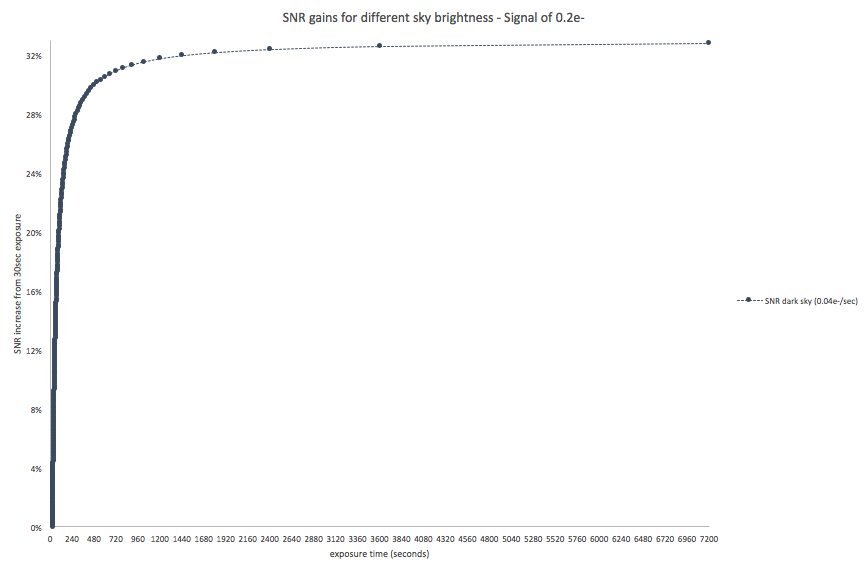
Please note that these gains are dependant on the signal coming from our target object as well. So if we would take a much fainter object with a flux of only 0.2e- compared to the 1e- we just saw, we get the following graph:
Wow! we can see the same strong curve with diminishing returns, but the SNR improvements for a fainter signal are clearly much much higher! Let’s look at the same minute marks as before: using 3.5 minutes exposures compared to 30sec exposures gives you an SNR improvement of 26.3%. Going from 3.5 minutes to 8 minutes gives you a further improvement of 2.86%. So even though we still see strong diminishing returns, the improvements remain significant up till longer exposures as before.
In this scenario, the improvement in SNR between 8 and 15 minutes still is 1%.
Please note that we’re talking about signal coming from the sensor here. So this includes scenarios with slow optics as well as using fast optics on very faint signal.
The role of read noise in SNR
To make it really clear what the role is of the read noise in the scenario’s we just ran through, let’s take a look at the role of read noise specifically and how it adds up when we add more exposures.
Let’s look at the SNR again for the same situation as described before, 12x10min subs versus 120x1min subs and rewrite it a bit just to see what is happening to the noise terms:
For the object shot noise, sky noise and dark current noise we get the following:
12x10min:
which is;
=
and for the 120x1min this then is;
=
So you see this is exactly the same, just as we could expect and have seen before in the scenario without read noise.
Now, let’s see what is happening with the read noise; which is simply
12x10min:
120x1min:
So the read noise is growing with the square root of the number of exposures in our integration, while all the other terms simply grow by total exposure time alone. So for a given fixed total exposure time, the read noise will be smallest with the least number of exposures.
Read noise is growing with the square root of the number of exposures in our integration
To see it’s impact in the total noise let’s run the actual numbers. Remember, uncorrelated noise adds up quadratically, so the total noise we get in these situations is;
12x10min:
120x1min:
Now we can clearly see how big the impact is of the read noise in this scenario.
Next let’s see what these numbers and the impact of read noise looks like in case of a bright sky. Let’s say sky background flux is 50e-.
For the time dependant noise sources we get:
=
If we add the read noise:
12x10min:
120x1min:
This is a totally different situation and the read noise could simply be considered irrelevant.
In fact, this looks very much like the hypothetical situation without any read noise we saw earlier.
So we can conclude that the exposure time only is relevant when the read noise is relevant. And the read noise is only relevant if the sky is dark enough.
The exposure time only is relevant when the read noise is relevant. And the read noise is only relevant if the sky is dark enough.
Determining optimal exposure time
Now we’ve seen the scenarios above you might wonder what would be applicable to your specific situation. As we just concluded this will be dependant mostly on the brightness of the sky you are imaging under.
There are formulas to determine the optimum exposure using the read noise and sky flux as input. There is also a script in PixInsight which you can use to give you an ‘ideal exposure length’. (Scripts->Instrumentation->CalculateSkyLimitedExposure)
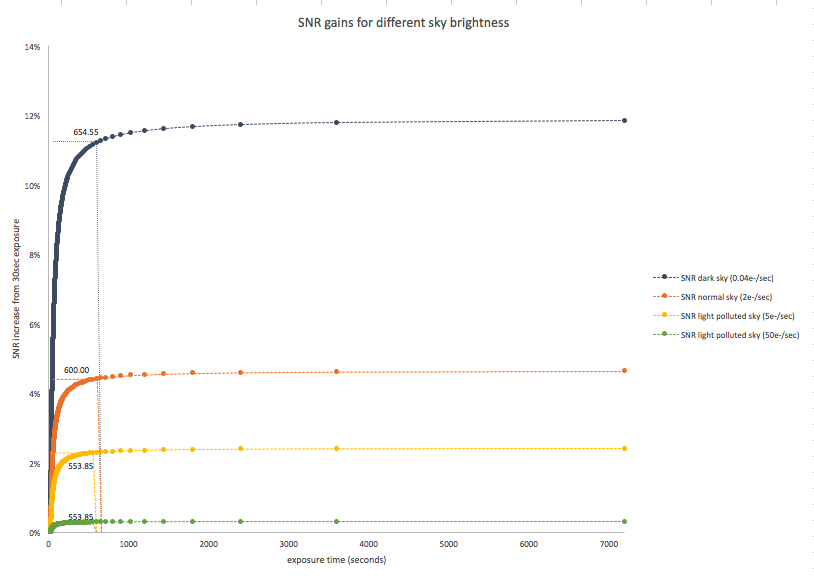
However, as we’ve seen in the graphs before the benefit in SNR is one of diminishing returns. This means it is not possible to give one absolute answer to the question what the optimal exposure time is. Assumptions need to be made about how much contribution of read noise to the total noise you will tolerate. And the differences in this assumption is often huge (factor 2 differences). Furthermore they don’t take practicalities into account. So I’d like to just show you a few more scenarios and the SNR corresponding gains for longer exposures compared to using 30sec exposures in a situation where we use a total exposure time of 2 hours. I’ve listed the ‘95% improvement mark’ for exposure time for each sky brightness.
The most obvious thing we can learn from this chart is that there is a huge difference how much you benefit from longer exposures under a dark sky versus brighter skies. Furthermore, the 95% improvement mark seems to be awfully close for all scenarios. However, I’m not sure how useful this number is since the next step up in exposure after this mark under a dark sky will still give you 0.05% increase while this improvement is only 0.0012% in the brightest scenario. To make this even more clear; for the brightest scenario the SNR for 30sec. exposure was 11.83, while all the way at the end with one exposure of 120 minutes the SNR was 11.86. So we could safely consider this a scenario where subexposure length is completely irrelevant.
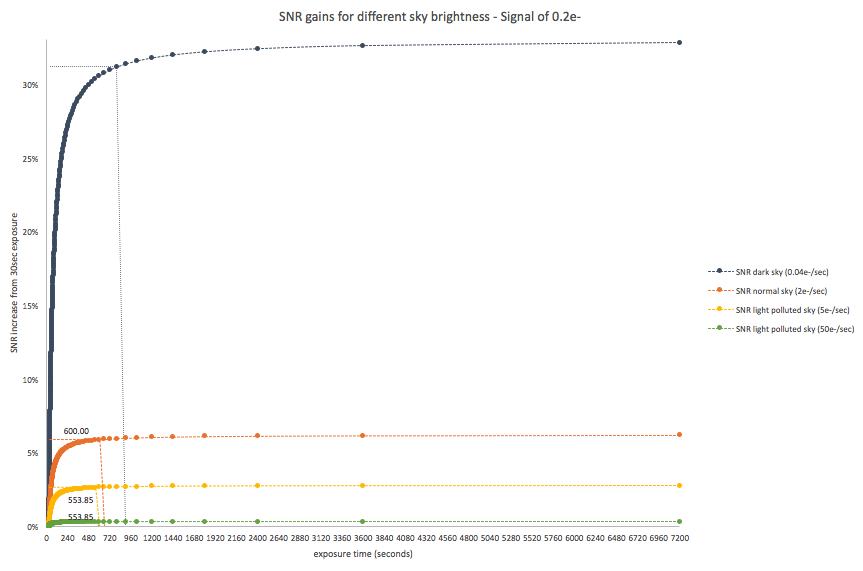
Remember we saw earlier that the SNR improvements were much larger if we are dealing with faint signals. So let’s look at the SNR improvements for different sky brightness with the previously used faint 0.2e- signal
The impact of the sky brightness is very clear again in this chart. Although we do benefit more from longer exposures when dealing with fainter signal also from brighter skies, the difference is negligible for very bright sky and is really huge for the really dark sky.
Based on these graphs we can conclude that if you are imaging under a light polluted sky you should not worry much about your exposure length. Every sky brightness between 5e- and 50e- basically has a total SNR improvement between 0.2% and 2% for which you’ll reach 80% of maximum SNR increase already at 3 minute exposures.
Other practical considerations to take into account
Next to the read noise and background sky flux, we need to take some practical factors into account as well when we want to determine the optimal exposure length. The ability to guide accurately for longer exposures for instance is of course really important, as well as the cost of loss of data if you need to throw out a subexposure. Remember that the total integrated exposure time is most important for SNR, so there is a real significant cost when you need to throw out subexposures.
Furthermore you might want to use (bayer) drizzle integration, which will need a minimum amount of (dithered) subexposures to give proper results.
These are all very important things to consider which might change things completely in terms of the optimal exposure time for you personally.
Light pollution and narrow band filters
I got asked a lot about the influence of filters. I haven’t tested this out myself, but the book The Astrophotography Manual covers a comparison between different light pollution filters and concluded that in his test it didn’t effect the background sky flux all that much (which was surprising). As always, test it out to know for sure, but I think the effect of light pollution filters is minimal on the read noise contribution to the overall noise.
Narrowband filters on the other hand will basically change even a light polluted sky to a dark(er) sky in general. So with narrowband filters you will benefit a lot from longer subexposures.
Of course there will always be exceptions to this, like OIII imaging with full moon, and exact details will depend on the speed of your scope and the quantum efficiency of your camera for instance. However, the generalisations will hold true in most situations.
A comparison from a dark site
Let’s look at the following data that I shot while I was in Namibia. There is practically no light pollution there and this was shot without the moon present. The only bit of background sky flux was the sky glow and perhaps a bit of zodiacal light.
The data was shot during 3 different nights so some variation is to be expected based on conditions for that particular night. There were no noteworthy differences between those nights so I have no reason to believe this is influencing the comparison much.
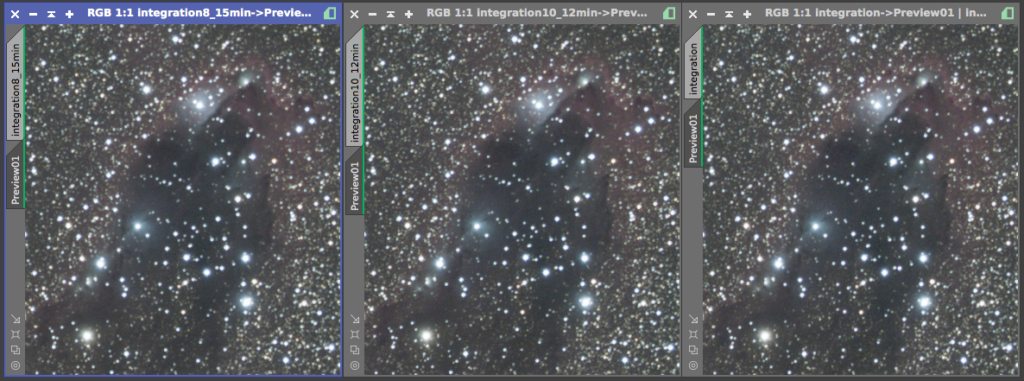
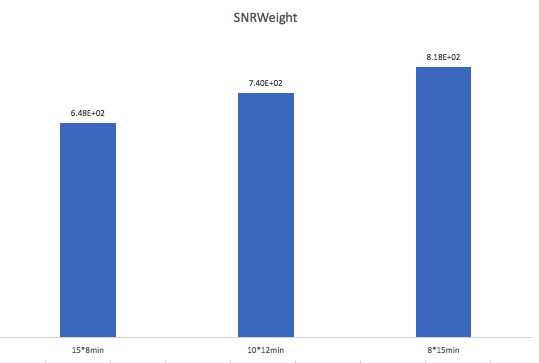
I had used different exposure lengths: 8, 12 and 15 minutes. For this comparison I made three integrations that all had the total integration time of 120minutes. So 15 frames of 8 minute exposures, 10 frames of 12 minute exposures and 8 frames of 15 minute exposures. No processing is done to these integrations. Just a STF applied to make the data visible.
Based on what we learned before we would expect the fewer but longer exposures will have the better SNR. Let’s see if this was the case:
Hard to tell from this wide shot, so let’s zoom in a bit.
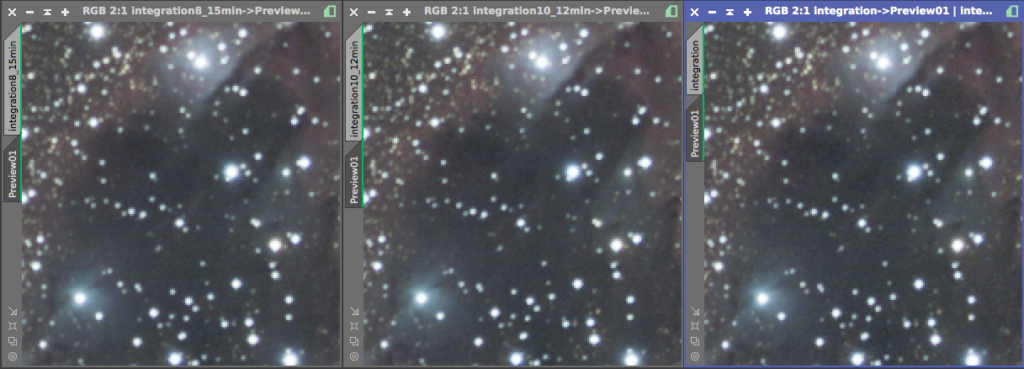
Here we can already see some difference in the amount of noise. Let’s zoom in further to have a better look.
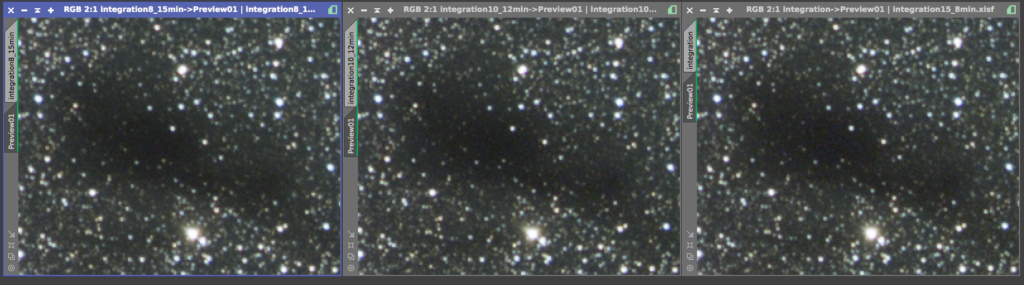
The differences in SNR are clearly visible now and we see indeed what we expected: the 8x15min. integration looks (much!) better than the 15x8min. integration.
This is even better visible in the dark region of Barnard 44A:
So visually we can already draw the conclusion that we were right: longer exposure has better SNR in this situation where we are clearly read noise limited. Now let’s check the numbers to confirm.
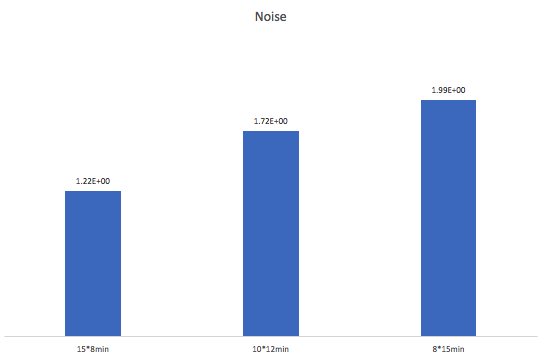
First let’s look at the amount of noise:
Noise is higher in longer exposures which is expected. The question is; did the signal grow more than the noise?
The SNRWeight measure (to be clear; this is a relative measure and not reflective of the actual SNR differences)
So yes, it clearly did.
Unless you are under dark skies, the subexposure length won’t matter much once you are using 2 to 3 minute exposures.
Conclusion and final considerations on sub exposure time and number of exposures
With all that we learned and the simulations we looked at and the real world dark site test we have quite some information about the optimal subexposure length. However, I bet you still are wondering how this impacts your particular situation and what the optimal subexposure length is. That will remain difficult to answer exactly, and much of the details go right out of the window if we need to take other factors like drizzle integration and guiding errors into account. However, I feel the most important conclusion probably is the fact that the exposure length is only relevant when the read noise is relevant. And the read noise is only relevant when you are imaging under a dark sky.
With most moderately to strong light polluted skies, the subexposure length won’t matter much once you are using 2 to 3 minute exposures.
Let me know in the comments below if you agree or disagree or still are left with questions at this point!


If you use short exposures 5-20″ you have another big advantage, small size stars…this means much resolutions
No, it just means that your tracking is no up to the task! Get a proper mount with a low PE and good guiding and you will get PIN POINT stars even with > 1800 secs exposures.
Well I partially agree. If you are under bad seeing conditions, long exposures will makes star bigger and object resolution lower.
But if the seeing is good, then, yes, it’s a matter of polar alignment and auto-guiding quality.
When exposures get to short wouldn’t you run into the issue of not utilizing your sampling resolution of your AD convertor?
When dealing with sufficient noise I don’t think that’s the case. Even with 3e- read noise you’ll be sufficiently dithering the ADC.
http://dslr-astrophotography.com/worry-unity-gain/
Interesting article and a wonderful site that I’ve only recently discovered. I do have a question. I have light pollution at my location. I have used a Light pollution filter to control it, and it does seem to do a good job because I don’t suffer from gradients. I was wondering how the use of a LPF impacts your calculations? I typically am shooting ISO 800-1600 with 600-900sec exposures. Does it give me a pseudo “dark sky” by removing the pollution?
The only thing that’s certain is that the LPF ‘shifts you towards darker sky conditions’. How much will depend on your personal equipment and situation.
However, if you are able to take 900sec exposures its quite likely you’ll benefit from these long exposures. But if you can I’d just take a 600sec exposure and 900sec and compare SNR so you can know for sure 🙂
Thank you for a great article.
What would you make of the BBC experiment a while ago, where they asked the public to take out their camera’ and make a photo of Orion’s belt. A great variation in light pollution, type of camera, exposure etc. AND a very value for N. Have a look at https://data.zooniverse.org/orion/all_stack_wide_step_number_1567_2.tif for the result
Thanks,
Leo
[…] quite extensive and mathematically sound treatment can be found here (while a more qualitative exposition is in Ashley) and these are its key […]
Great article. Very enlightening (!) Seriously, it’s nice to get a scientific, math-based take on this much discussed topic. Thank you!
[…] C.V. Longer exposures versus multiple shorter exposures. December 10, 2016. Disponível em: <https://dslr-astrophotography.com/long-exposures-multiple-shorter-exposures/>. Acesso em: […]
Excellent article with 1 minor nit to pick:
“Please note that we’re talking about signal coming from the sensor here. So this includes scenarios with slow optics as well as using fast optics on very faint signal.”
The factor Sky_s very much depends on your optics. It is the sky flux as measured on the photosite. If I use faster optics I’m gathering more signal but I’m also gathering more sky flux at the ratio.
In my personal setup, I can compare my old Esprit 80ED and my newer RASA8. They have about the same FL but the RASA8 gathers about 4x more light (when subtracting the central obstruction). The sky flux numbers I measure in my background are also roughly x4 when using the same camera exposure and settings.
Děkuji za super článek.Chci fotit PaStDr 9 s Canon 450Da a N135/550.Mám použít Optolong L-eNhance nebo bez něho..Děkuji
Finally an article that provides the real arguments and math to the dilema of lots of shorts versus few longs. “The read noise is different in the sense that this doesn’t build up over time but it does occur once for every exposure”, that’s the key piece I wanted to understand, this non-affected-by-exposure-time factor that makes the difference (I do untracked astrophotography). Thanks!
Glad the article was useful for you!
Very nice article, congrats. This reaffirms my thoughts.
One question; which camera did you use when shooting from Namibia?
That is a good tip especially to those fresh to the blogosphere.
Simple but very accurate information… Thank you for sharing this one.
A must read post!